Dealing with Notations and conventions in tensor analysis-Einstein's summation convention covariant and contravariant and mixed tensors-algebraic operations in tensor symmetric and skew symmetric tensors-tensor calculus-Christoffel symbols-kinematics in Riemann space-Riemann-Christoffel tensor.

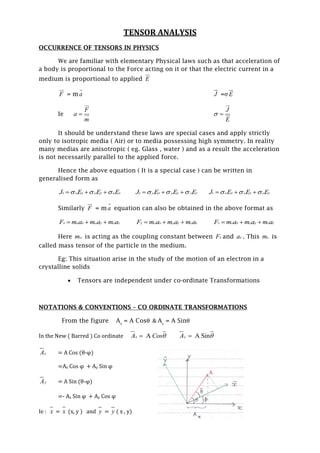
1. TENSOR ANALYSIS
OCCURRENCE OF TENSORS IN PHYSICS
We are familiar with elementary Physical laws such as that acceleration of
a body is proportional to the Force acting on it or that the electric current in a
medium is proportional to applied E

F

= m a

J

=σ E

Ie
F
a
m



J
E
 


It should be understand these laws are special cases and apply strictly
only to isotropic media ( Air) or to media possessing high symmetry. In reality
many medias are anisotropic ( eg. Glass , water ) and as a result the acceleration
is not necessarily parallel to the applied force.
Hence the above equation ( It is a special case ) can be written in
generalised form as
xx xy xzx x y zJ E E E     yx yy yzy x y zJ E E E     zx zy zzz x y zJ E E E    
Similarly F

= m a

equation can also be obtained in the above format as
xx xy xzx x y zF m a m a m a   yx yy yzy x y zF m a m a m a   zx zy zzz x y zF m a m a m a  
Here xxm is acting as the coupling constant between xF and xa . This ijm is
called mass tensor of the particle in the medium.
Eg: This situation arise in the study of the motion of an electron in a
crystalline solids
 Tensors are independent under co-ordinate Transformations
NOTATIONS & CONVENTIONS – CO ORDINATE TRANSFORMATIONS
From the figure Ax
= A Cosθ & Ay
= A Sinθ
In the New ( Barred ) Co ordinate AxA Cos A SinyA 
xA = A Cos (θ-φ)
=Ax Cos φ + Ay Sin φ
yA = A Sin (θ-φ)
=- Ax Sin φ + Ay Cos φ
Ie : x = x (x, y ) and y = y ( x , y)

2. Consider an N dimensional space and let x1,x2……….. xN be any set of co-ordinate in this space.
Similarly 1 2
.................., , ,N
x x x be another set of co-ordinate in the same space . ie xi is the old
coordinate system where i(1≤i≥N) and x
(1≤∝≥N) be another set of co ordinate in the same
space.
Then from above concept we can write
1 1 1 2
..............( ., , ... )N
x x x x x 2 2 1 2
..............( ., , ... )N
x x x x x
1 1
x x ( x1,x2…………..….. xN ) 2 2
x x ( x1,x2…………..….. xN )
In general 1 2
............( , ..... ), .i i N
x x x x x (1≤i≥N)
x x 
 ( x1,x2…………..….. xN ) (1≤∝≥N)
The above two equations defines the coordinate transformations. On differentiating equation
(1)
1 1 1
1 21
1 2
.........
N
N
x x x
dx d x d x d x
x x x
  
   
  
1 2
1 2
.........
i i i
Ni
N
x x x
dx d x d x d x
x x x
  
   
  
1 1 1 1
1 1 2 3
1 2 3
..... N
N
x x x x
d x dx dx dx dx
x x x x
   
    
   
1 2 3
1 2 3
..... N
N
x x x x
d x dx dx dx dx
x x x x
   
    
    
   
1
iN
i x
dx d x
x


 



 (1≤i≥N)
1
N
i
i
x
d x dx
x


 



 (1≤∝≥N)
EINSTEIN SUMMATION CONVENTION
If any index is repeated in a term then a summation w.r.t that index over the range 1,2,3
…………N is implied. This convention is called Einstein’s Summation Conevention.
According to this convention, instead of expressing
1
n
i
 aixi we merely write aixi hence
equation (2) can be written as
i
i x
dx d x
x





(1≤i≥N) i
i
x
d x dx
x

 


(1≤∝≥N)

3. Thus the summation convention means the drop of sign for the index appearing twice in a given
term. In other words the Summation Convention implies the sum of the term for the index
appearing twice in that term over a define range.
The index ∝ occurs twice on the RHS of equation (3a) while I occurs twice on the RHS
(3b) summation over these from 1 to N is therefore implied in the respective equations.
 If an index appears only once in any term , it has a definite value any value
between 1 and N such an index is called a free index
 Hereafter the specification such as 1≤i≥N will be dropped and hence (3a)
becomes
i
i
x
d x dx
x

 


i
i x
dx d x
x





An index which is repeated and over which summation is implied is called a dummy index. A
dummy index can be replaced by any other index which doesn’t appear in the same term.
SPECIAL CASE
It is clear that from the above theory , On squaring of aixi is
= (aixi)( aixi) ------------------------------------------------wrong concept
= aixi . ajxj ……………………………………………………………………………………….. True concept
Here LHS = (a1x1 + a2x2 ) (a1x1 + a2x2 )
= a1x1. a1x1 + a1x1. a2x2 + a2x2. a1x1 + a2x2. a2x2
= aixi . ajxj ≠ aixi . aixi
KRONECKER DELTA
We define the Kronecker delta as δi
j or δij
1ij  for i=j
0ij  for i≠ j hence the Kronecker delta can be consider as a dot product of
two unit vector.
(1) .ij i j  , let us consider two vector a

= ˆia i & b

= ˆjb j
a

.b

= ˆia i . ˆjb j = i ja b ˆ ˆ.i j = i ja b ij
When a

 b

 a.b = 0 ie here 0ij  for i≠ j
When a

 b

 a.b =ab ie here 1ij  for i= j
(2) The Kronecker delta j
i can be written as j
i =
j
i
x
x


= 1 when i=j &
j
i =
j
i
x
x


= 0 when i≠ j
(3) Kronecker delta is a tensor quantity ie , in 3 Dimension ij = 3 & in Phase Space ij =
6N
(4) Kronecker delta is Symmetric ie ij = ji since . .j i i j

4. (5) We know that
i
i
j j
x
x




or
i
ij j
x
x




i i
i
j j j
x x x
x x x



  
 
  
= i
j

   i
j = i
j

 
Or i
j = i j   , here in statement no (5) , ∝ is a dummy index and I & j are free
indices.
Hence
i
i
j j
x x
x x



 

 
or
k
k
x x
x x


 

 

 
When we undergo transformation the component of a tensor changes by two different
ways
1. Contravariant Tensors
2. Covariant Tensors
CONTRAVARIANT VECTORS
Let us consider a displacement vector
x x 
 ( x1,x2…………..….. xN )
1 1 1 1
1 1 2 3
1 2 3
..... N
N
x x x x
d x dx dx dx dx
x x x x
   
    
   
i
i
x
d x dx
x

 


 i
i
x
A A
x

 


A

are the component of vectors like component in the new coordinates
system . i
x
x



is the transformation coeffient .
•Let a physical entity characterised by the N functions A when expressed in the
x coordinate system .When we measure the same entity in coordinate system
,characterised by the components a.The are said to be the component of a
contravariant vector if they transform under coordinate transformation .
i
i
x
A A
x

 


Inverse transformation is obtained by multiplying
J
x
x



J J
i
i
x x x
A A
xx x


 
  

 
{ for multiplication we use
j
x ( j is free index in LHS & RHS) x

 ( ∝ is dummy index in
LHS & RHS )}
=
J
i
i
x x
A
xx


 

= δj
i
i
A  j
A

5. j
A =
J
x
A
x




{ The indices must shows the same properties in LHS & RHS )
COVARIANT VECTORS
A set of N quantities Ai
which are functions of i
x coordinate system are said to
be the component of a covariant tensor if they transform
i
iA
x
x
A



Under a change of coordinate from i
x to x

where A are the component of a
vector in the barred coordinate system .
→ Consider a scalar gradient  in two coordinate system
x1,x2…………..….. xN
1 2
................, , .. N
x x x
1 2 3
, ,
x x x
    
   1 2 3
, ,
x x x
    
  
1 2 3
, ,
x x x
    
  
(since is scalar  =  )
1 2
1 21 1 1
x x
x xx x x
      
 
   
+………….+
1
N
N
x
x x
 
 
In general
i
i
x
xx x 
   

 

i
i
x
xx x 
   

 
i
iA
x
x
A



Inverse transformation obtained by Multiplying j
x
x



i
j j iA
x x
A
x x x
x
 

  

  
= i
j iA = jA jA = j
A
x
x





6. TENSOR OF RANK TWO
A set of N functions are said to be the component of a contravariant tensor of
rank 2 ,if they transform .
ij
i j
x x
A A
x x
 
  

 
In general no. of component = Nr
[ N – dimension r – rank ]
i j
ij
x x
A A
x x

 
 

 
MIXED TENSOR OF RANK TWO
j
i
j
i
x x
A A
x x




 

 
Eg: kronecker delta
It can be represented ij , ij
 , i
j as in Cartesian coordinate system .since
there is no distinction between covariant and contravariant tensors in
rectangular coordinate system .
But its true value is i
j
ij Concept is true in rectangular coordinate but false in general theory of
relativity and Riemanian space
TENSORS OF HIGHER RANK
The rank of a tensor only indicate the number of indices attached to it per
component .Eg: ij
kA are the component of a mixed tensor of rank 3 having
contravariant rank 2 and covariant rank 1 .if they transform according to
equation
k
ij
k
i j
x x x
A A
x x x
 



  

  
The number of component of rank is given by Nr
SYMMETRIC TENSORS
If two contravariant or covariant indices can be interchanged without altering
the tensor ,then the tensor is said to symmetric with respect to these two
indices .
ij ji
A A ij jiA A

7. For a tensor of higher rank ijk
lA if ijk jik
l lA A is said to be symmetric w.r.t the
indices i,j only .
 symmetric property is independent of the coordinate system used .
 A symmetric tensor of rank 2 in N-dimensional space has
( 1)
2
N N 
independent component
Eg : moment of inertia about XY axis is equal to YX axis .
xx
y
xy xz
yy yz
zy z
x
x zz
I
I
I
I I
I I
I I
 
 
 
 
 
It is a second rank tensor with n2
(32
=9 ) components out of which n (3)
components are diagonal and rest n2
-n (9-3=6) will be in equal pairs due to
symmetry.
Total number of component = n( diagonal ) +
2
2
n n
= n +
2
2
n n
=
2
2
2
n nn 
=
( 1)
2
n n 
In general number of component of a rank symmetric tensor will be
( 1)!
!( 1)!
n r
r n
 

Case 1 , When r=2 ,
( 2 1)!
2!( 1)!
n
n
 

=
( 1)!
2( 1)!
n
n


=
( 1)
2
n n 
Ie.,the above result
SKEW SYMMETRIC TENSORS
A tensor ij
A or ijA is said to be skew symmetric (anti symmetric) if its element
satisfy
ij ji
A A  ij jiA A 
For a tensor of higher rank
ijk ikj
l lA A  ,then the tensor ijk
lA is skew symmetric w.r.t indices j and k
 if a tensor is such that two contravariant or covariant indices of it when
interchanged, the component of the tensor alter in sign, not in magnitude
the tensor is skew symmetric
 the property of symmetric is an intrinsic property of a tensor and is
independent of the choice of coordinate system

8.  symmetry and antisymmetry can only be defined for a pair of similar
indices
 Symmetric in the first two contravariant indices. It is important to specify
the position of the indices rather than the indices themselves
 Symmetry and antisymmetry can be defined only for similar indices not
when one index is covariant and the other is contravariant.
TENSOR ANALYSIS
1. EQUALITY AND NULL TENSOR
Two Tensors are said to be equal iff they have the same ( number&
type ) contravariant and covariant rank and every component of one is equal to
the corresponding component of the other.
1 2 3
1 2 3
, , ,
, , ,
p
p
i i i i
j j j jA

 = 1 2 3
1 2 3
, , ,
, , ,
p
p
i i i i
j j j jB


If all the Nr
components of a tensor of total Rank r identically vanish , It is
said to be a null tensor.
2. ADDITION & SUBTRACTION
The sum and difference of two or more tensors of the same rank results in a
third tensor of the same rank .Moreover if Fλµ =Gλµ and are tensors of the same
rank the is also a tensor of same rank .
ij ij ij
k k kA B C 
To add or subtract any two tensors corresponding elements are added or
subtracted
ij ij ij
A B C  
11 12
21 22
a a
a a
 
 
 
+
11 12
21 22
b b
b b
 
 
 
=
11 11 12 12
21 21 22 22
a b a b
a b a b
 
 
 
 
 
( ) ( )( )
j
i i
j ji
x x
A B A B
x x

 
 
 
  
 
( )
j
i
ji
x x
C C
x x


 
 

 
Statement –
Any tensor having either two contravariant or covariant indices can be
expessed as a sum of two parts one symmetric and the other is skew symmetric
Let ij
A being any tensor then we can write

9. ij
A =
1 1
( ) ( )
2 2
ij ji ij ji
A A A A  
= ij
B + ij
C
{we wanted to prove that is ij
B symmetric and ij
C is antisymmetric so that ij
A
can be represented as = symmetric tensor + antisymmetric tensor }
ij
B =
1
( )
2
ij ji
A A , ---(1) On interchanging the indices
ji
B =
1
( )
2
ji ij
A A which is same as (1) hence ij
B = ji
B
ij
C =
1
( )
2
ij ji
A A ----(2)
ji
C =
1
( )
2
ji ij
A A = -
1
( )
2
ij ji
A A = - ij
C shows that it is skew symmetric
3. OUTER PRODUCT
The Outer product of the two tensor is a tensor whose rank is the
sum of the ranks of the given tensor. Thus if r and q are the ranks of two
tensors , thus the outer product will be a tensor of rank (r+q).
The tensors Aij
k
and BP
q
transform according to following equation
k
ij
k
i j
x x x
A A
x x x
 



  

  
&
q
p
q
p
x x
B B
x x




 

 
Multiplying the components
qk
ij p
k q
i j p
x x x x x
A B A B
x x x xx
  
 
 
 
    

   
Let A B
 
  = C


ij p
k qA B = ijp
kqC
qk
ijp
kq
i j p
x x x x x
C C
x x x xx
  


 
    

   
Cijp
kq
is a tensor of contravariant Rank 3, covariant Rank 2 and total Rank 5. It
has therefore N5
components each of which is the product of Aij
k
and BP
q
This is known as the outer product or Kronecker product of two tensors.
The concept of outer product of tensors can be easily extended to more
than two tensors. The contravariant rank of the outer product tensor is the sum
of the contravariant ranks and the covariant rank is the sum of the covariant
ranks of the tensors whose outer product it is.

10. 4. INNER PRODUCT OF TENSORS
Let us consider two tensors Aij
k
and BP
q
consider a set of functions
Aij
k
and BP
q
where it has three free indices, there will be N3
functions. We wanted
to show that N3
functions are the components of a tensor of Rank 3.
For this purpose put ρ = γ in equation (1)
qk
ij p
k q
i j p
x x x x x
A B A B
x x x xx
  
 
 
 
    

   
qk
ij p
k q
i j p
x x x x x
A B A B
x x x xx
  
 
 
 
    

   
q
k ij p
k q
i j
x x x
A B A B
x x x
 
 
  


  

  
Now P is a dummy index. Owing to the appearance of the delta symbol it is clear
that the summation over P from 1 to N , only that term will survive for which
p=k
q
ij k
k q
i j
x x x
A B A B
x x x
 
 
 

  

  
This transformation coefficient shows that the tensor transforms like the
components of a tensor of contravariant rank 2 and covariant Rank 1. Now let
us denotes the component of a New tensor as
C A B
  
   & ij ij k
q k qC A B
q
ij
q
i j
x x x
C C
x x x
 



  

  
Then the Cij
q
is said to be the inner product of the two tensors Aij
k
and BP
q
.
Aij
k
and BP
i
& Aij
k
and BP
j
are the inner product of Aij
k
and BP
q
. In taking the
inner product of two tensors it is important that one contravariant index of
tensor should be equated to one covariant index of the other.
CONTRACTION OF TENSORS
The algebraic operation in which the rank of a tensor is lowered either by
two or multiple of 2 is known as contraction. In this process of contraction one
contra variant index and one covariant index of a mixed tensor are set equal ,
repeated index summed over , the result is a tensor of rank lower by 2 than the
original tensor.
l m
ijk
lm
i j k
x x x x x
A A
x x x x x
  


 
    

    

11. Putting ρ = α in the above equation and summing over α from 1 toN
l m
ijk
lm
i j k
x x x x x
A A
x x x x x
  


 
    

    
.
m
l ijk
i lm
j k
x x x
A A
x x x
 




  

  
m
ijk
im
j k
x x x
A A
x x x
 



  

  
This shows that Aijk
im
is a tensor of contravariant rank 2 and covariant rank 1.
This process is known as the contraction of a tensor. When a tensor is
contracted by equating one of the contravariant indices with one of its covariant
indices , the resulting entity is a tensor whose rank contravariant and covariant
ranks reduced by one each. Thus reducing the total rank by two.
Aijk
li
, Aijk
jm
, Aijk
lk
are various contracted forms of the tensor Aijk
im
. A tensor
can be repeatedly contracted , thus a tensor Aijk
lm
of total Rank 5 , on contraction
gives a tensor Aijk
im
of total rank =3 , which can be further contracted to gice the
tensor Aijk
ij
or Aijk
ik
of contravariant Rank 1.
QUOTIENT LAW
Quotient law states that if the inne product of an entity with an arbitrary
tensor is a tensor , the entity is a tensor
Let us consider the entity having N3
functions, A (i, j, k). Suppose it is
knownthat the inner product of A (i, j, k) with an arbitrary tensor Bjk
is
contravariant tensor of Rank 1
A (i, j, k) Bjk
= Ci
, ‘i’ is the free indices . Summation over j and k on the
LHS is implied.
Let A (α,β,γ) be the N3
functions in the barred co ordinate system, then
A (α,β,γ) B βγ = C α
A (α,β,γ) jk i
j k i
x x x
B C
x x x
  
  

  
= i
x
x



A(i,j,k)Bjk
{ Since Bjk is a contra variant tensor with rank = 2 & Ci is a contra variant }
[ A (α,β,γ) j k
x x
x x
 
 
 
- i
x
x



A(i,j,k) ] Bjk = 0 , this equation must be true for an arbitrary
tensor Bjk , expression in the square bracket = 0
A (α,β,γ) j k
x x
x x
 
 
 
= i
x
x



A(i,j,k) , then by taking inner product with
j k
x x
x x
 
 
 
in LHS &
RHS of the above equation

12. A (α,β,γ)
j k
x x
x x
 
 
 
j k
x x
x x
 
 
 
=
j k
x x
x x
 
 
 
i
x
x



A(i,j,k)
A (α,β,γ)



 =
j k
x x
x x
 
 
 
i
x
x



A(i,j,k)
A (α,β,γ) =
j k
i
x x x
x x x

 
  
  
A(i,j,k)
which shows A (i, j, k) is a tensor of contravariant rank 1 and covariant rank 2
which can be written as Ai
jk
j k
i
jk
i
x x x
A A
x x x



 
  

  
 It is important in the use of the quotient law that the tensor with which the inner
product is taken should be an arbitrary tensor
FUNDAMENTAL TENSOR
Easier to handle Cartesian tensor than general tensor , because in
Cartesian there is no distinction is made between covariant and contravariant .
But in General theory of relativity , the presence of Gravitational field produce
curvature in the space time continuum and thus the Euclidean character of the
space is destroyed.
{ Small space ds , It is very small may be regarded as flat space , If we look from a longer
distance It is Curved }
Consider a region ds
Cartesian Tensor = f( General Tensor )
Xi
= Xi
( x1
,x2
, x3
)
dS2
= dX(1)2
+ dX(2)2
+ dX(3)2
= ( dXi
)2
= dXi
dXj
=
i i
X X
dx dx
x x
 
 
 
 
= gαβ dx dx 

13. gij
tells the nature of Space .
Fundamental metric Tensor is an expression which expresses the
differences between two points – metric
ds2
= gij
dxi
dxj
, If we change i & j ds2
= gji
dxj
dxi
which means
gij
= gji
ie It is Symmetric
here ds2
is a scalar quantity formed by multiplying ( gij
dxi
) with dxj
( gij
dxi
).dxj
----------------------- Scalar [ Vector. Vector = Scalar ]
( gij
dxi
).-------------- Vector = Covariant Vector hance gij
is a covariant
tensor with Rank 2.
Euclidean space means flat space and Riemannian Space means Curved
Space . If all the coefficient of gij
is independent of xi
space becomes Euclidean
Space .
gij
dxi
dxj
g dx dx
 
i j
i j
x x
g dx dx
x x

 
 
 
= gij
dxi
dxj
[ ] i j
ij
i j
x x
g g dx dx
x x

 
 

 
= 0
ij
i j
x x
g g
x x

 
 

 
multiplying by
i j
x x
x x
 
 
 
we get
i j
ij
x x
g g
x x

 
 

 
CONTRAVARIANT FORM OF FUNDAMENTAL TENSOR
Let us introduce the contravariant form of fundamental tensor
gij
=
1
g
Cofactor of gij
Consider the inner product of fundamental metric tensors
gij
.gkj
= gi1
.gk1
+ gi2
.gk2
+ gi3
.gk3
+ ………….
=
1
g
{ gi1
.cofactor of gk1
+ gi2
.cofactor of gk2
+ ……………..
But gi1
x cofactor of gi1
= g and gi1
x cofactor of gk1
= 0 if i≠k
Hence gij
.gkj
=
1
g
x 0 when i≠k

14. =
1
g
x g when i=k
ASSOCIATE TENSORS
Let Ai
be an arbitrary contravariant vector . The inner product of Ai
with
the covariant metric tensor gij
will be a covariant vector . this inner product is
denoted by
Ai
gij
= Aj
& Aj
gij
= Ai
, Showing that the relation between Ai
& Aj
is
reciprocal. The Tensors Ai
& Aj
are called Associate Tensors .
The Raising or lowering of indices of a tensor changes a covariant
index into a contravariant one and vice versa. The Operation depend on the
inner product of the given tensor with fundamental tensor
RAISING & LOWERING OF INDICES
When the inner product of a tensor is taken with gij
, one contravariant
index of the tensor is lowered to a covariant position .
Aij
. gjk
= Ai
-k
Aij
. gik
= Aj
k-
The Reverse process in which the inner product of a tensor is taken with
the contravariant metric tensor gij
raises a covariant index to a contravariant
position hence is known as the Raising of an index.
Ai
-k
gkj
= Aij
Ai
k-
gkj
= Aji
TENSOR CALCULUS
The partial derivative of a scalar field w.r.t the co ordinates are the
components of a covariant vector .
 The differentiation of a tensor ( except that of Rank = 0 ) w.r.t the co
ordinates doesn’t give a tensor.
Let us introduce ,
i
i x
x
x
 



,i i
x
x
x

 


2
,
i
i x
x
x x
  


 
,i i
x





, ...
.... ,
ij
ij k
k l l
A
A
x



1. DIFFERENTIATION OF A TENSOR
Let us consider a covariant transformation
i
iA
x
x
A



 ,
i
iAxA  

15. Differentiation both side of the above equation with x

2
,
i i
i
i
x x A
A A
xx x x
     
  
 
  
, , ,
i
i i
i
x A
A x A
xx
    
 
 

, , , ,
i i
i iA x A x A     
, , , , ,
i i j
i i jA x A x x A      {
j
i i
j
A A x
x x x 
  

  
, The second term on RHS has a
tensorial character but the appearance of the I term shows that the function ,i jA
do not transform like the component of a II Rank tensor
 The Co-ordinate derivative of any tensor ( Excluding the Scalar ) donot
transform like the component of a tensor
CHRISTOFFEL SYMBOLS
In Cartesian the difference of two vectors situated a two different point
of the space is a vector. But in Reimannian space Transformation equation vary
from point to point . Therefore the difference of two vectors are considered at
the same point of the space.
Thus to find the difference of the two vectors in Riemmannian Space one
of the two vector is to be parallel displaced to the point of location of the other.
 In Cartesian system parallel displacement donot change any magnitude .
But in Riemannian space ( curved ) components are changed due to
parallel displacement.
 So consider a Physical quantity Aμ at a point with co- ordinate xμ . When it
is displaced parallel to neighbouring point with co-ordinate
xμ + dxμ Aμ +
A

A
 0 in cartesian co-ordinate ( flat space )
≠ 0 in curved space
Thus A
 = 
 A dx 
Where 
 vanish in flat spce . But exist in curved space. The Coefficint ( 
 )
are non Tensors.
Let us introduce the Christoffel three index symbol or simply Christoffel
Symbol of I & II Kind respectively by
, , ,
1
[ , ] ( )
2
ik j jk i ij kij k g g g  
[ , ]k km
ij g ij m  = , , ,
1
( )
2
km
im j jm i ij mg g g g 
PROPERTIES
1. [ , ]ij k = [ , ]ji k
Since , , , , , ,
1 1
( ) ( )
2 2
ik j jk i ij k jk i ik j ji kg g g g g g    
But these two are equal ij jig g & kj jkg g

16. 2. k k
ij ji  
LHS = [ , ]k km
ij g ij m 
RHS = [ , ]k km
ji g ji m  but [ , ]ji m = [ , ]ij m , Hence k k
ij ji  
3. ,ij kg = [ , ] [ , ]ik j jk i
RHS = , , , , , ,
1 1
( ) ( )
2 2
ij k kj i ik j ji k ki j jk ig g g g g g    
= , ,
1
( )
2
ij k ji kg g = ,
1
(2. )
2
ij kg = ,ij kg = LHS
TRANSFORMATION OF CHRISTOFFEL SYMBOL – I KIND
ie [ , ]  [ , ]ij k
,, ,
1
( )
2
g g g      [ , ]ij k
But we know that , ,
i j
ijg x x g  
Consider ,g 
,g  = , , , , , , ,[ ]i j i j i j
ij ijx x x x g x x g       
= , , , , , , ,[ ]i j i j i j k
ij ij kx x x x g x x x g       
Then similarly we can write ,g  & ,g 
,g  = , , , , , , ,[ ]i j i j j k i
ij jk ix x x x g x x x g       
,g  = , , , , , , ,[ ]i j i j i k j
ij ik jx x x x g x x x g       
In equation (1) I term of RHS we put i w.r.t ∝ and j w.r.t β. Consider RHS the
I term contain metric tensor ijg Symmetric. So it is not necessary to change
i j
Then from LHS = , , ,
1
( )
2
g g g      
Ie
1
2
{(3)+(2)-(1)} = , , , , , ,
1 1
[ ] { .2.[ , ]}
2 2
i j i j i j k
ijx x x x g x x x ij k       
= , , , , , ,
1
[ ] { .[ , ]}
2
i j i j i j k
ijx x x x g x x x ij k       
This is the transformation of Christoffel symbol of the I kind from one co-
ordinate system to another.
The presence of the I term on the RHS of the above equation shows that the
Christoffel Symbol of I Kind is not a Tensor.

17. TRANSFORMATION OF CHRISTOFFEL SYMBOL – II KIND

 m
ij
[ , ]g

  [ , ]mk
g ij k
the LHS is obtained by taking inner product of equation (A) with g

= [ , ]g

 
= , , , ,{ .[ , ]}i j i j k
ijg x x g x x x ij k

    
= , , , , . [ , ]i j i j k
ijx x g g x x x g ij k
 
     , to make g

in unbarred co-ordinate system
= , , , ,, , , , [ , ]i j lm i j k no
l m n oijx x x x g g x x x x x g ij k
   
    
= , ,, , , , , [ , ]i j lm i j k no
l nm ij ox x g g x x x g ij k
 
    , {Since ,
j lm lj
m ij ijg g g g   exist only when i=j }
= , ,, , , [ , ]i i j nk
i nx x x x x g ij k
 
  
= , ,, , ,
i i j n
i n ijx x x x x
 
   
Due to the presence of I term on RHS this is also not having tensorial in
character.
COVARIANT DERIVATIVE
It has been pointed out that space derivative of a scalar field is a vector.
Derivative of a scalar field transforms like Covariant Tensor . Eg : Gradient (∇φ )
i
iA
x
x
A



 ,
i
iAxA  
Differentiation both side of the above equation with x

, , , , ,
i i j
i i jA x A x x A     
Which is not a transformation equation as ,
i
x which destroys the tensor
character. However in Cartesian frame ,
i
x =0 .
∴ Above equation become , , , ,
i j
i jA x x A   
If ,
i
x = 0 ie vector ( Tensor with Rank = 1) derivative transformlike a covariant
tensor of Rank = 2 in Cartesian frame.
 In case of general curvilinear co ordinates , ordinary derivatives given by
(1) doesn’t transform as a tensor. So let us introduce a new kind of
derivative defined as ‘’ covariant derivative ‘’ specially for tensors.
, , , , ,
i i j
i i jA x A x x A      (1)
But from the transformation of Christoffel symbol II Kind
, ,, , ,
i i j n
i n ijx x x x x
  
      
On taking the inner product with k
x
, ,, , ,{ }k k i i j n
i n ijx x x x x x x
  
       

18. = , , , , ,
i k i j k n
i n ijx x x x x   
= , , , ,
i k i j k
i ijx x x x   
, , ,
k k i j k
ijx x x x

      
, , ,
i i i j k
ijx x x x

      
Substituting (4) in A
, , , , ,
i i j
i i jA x A x x A     
= , , , , ,{ }i i j k i j
ij i i jx x x A x x A

       
= , , , , ,
i j k i j
ij i i jA x x A x x A

       
, , , ,( ) ( )i j k
i j i ijA A x x A A

        
, , , ,( ) ( )i j k
i j k ijA A x x A A

        
The function ;i jA is called jth
covariant derivative of the vector iA
COVARIANT DERIVATIVE OF A CONTRAVARIANT TENSOR
Let us consider a contravariant tensor Ai
, The transformation equation given by
i
i
x
A A
x

 


---------------- --------------- ,
i
iA x A
 

, , , , ,
i j i
i i jA x A x x A
  
   
= , , , , ,
j i j i
ij i jx x A x x A 
 
[Substitution is made for converting ,ix
 to ,ijx
] , from previous session we proved that
, , ,
k k i j k
ijx x x x

       , But we want ,ijx
so put k--∝ , ∝-i and βj
, , , ,
k
ij k ij i jx x x x
   
    { here we put ρk , i- β and jγ }
, , , ,
k
ij k ij i jx x x x
   
   
From (b) & (c) , , , , , , ,{ }k j i j i
k ij i j i jA x x x x A x x A
    
      
= , , , , , ,
j k i j i j i
k ij i j i jx x A x x x A x x A
   
     
By combining I and III term , Put k=i & i by k
, , , , , , ,
j i j i j i k
i j i j i kjA x x x A x x A x x A
    
        
, , , ,[ ]j i i k
i j kjA A x x A A
   
     
, , , ,( ) ( )j i i k
i j kjA A x x A A
   
     
RIEMANN CHRISTOFFEL TENSOR
Consider an infinitesimal parallelogram PQRS as described with adjacent
sides PQ = dxi
and PR = δxi
. Imagine a contravariant vector i
A defined at a point
P ( i
PA )being parallel displaced in the following two ways.
1. Displace i
A parallel from P to Q resulting in i
QA & then displace i
QA
parallel from Q to S giving the vector i
SQA

19. 2. Displace i
PA parallel from P to R giving i
RA and then displace i
RA parallel
from R to S resulting the vector i
SRA
Now to find the two vectors i
SQA and i
SRA at S be equal . If not what
does the distance between them depends . Displace i
PA parallel from P to Q.
i i i
Q P PA A dA  = P
i i j k
P jk P PA A dx 
i i i
SQ Q QA A A  = P
i i l m
Q lm Q QA A x 
The Christoffel symbol depend on the metric
tensor which in turn is a function of co –
ordinates. For a small displacements
PQ = i
dx we can write
,Q P P
i i i n
lm lm lm ndx     Hence
,( )P P
i i i i n l m
SQ Q lm lm n Q QA A dx A x    
=
,( )( )P P P
i i j k i i n l l j k m
P jk P P lm lm n jkA A dx dx A A dx x       
= , ,
i i j k i l m i l n m i l j k m i l n k m
jk lm lm n lm jk lm n jkA A dx A x A dx x A dx x dx dx x              
Now i
SRA is obtained by just replacing dx by x and x by dx
i
SRA = , ,
i i j k i l m i l n m i l j k m i l n k m
jk lm lm n lm jk lm n jkA A x A dx A x dx A x dx x x dx               
The first 3 terms in the RHS of (1) & (2) are equal . ie in II and III term just
replace k by m Hence (2)- (1)
i
SRA - i
SQA = , ,
i l n m i l n m i l j k m i l j k m
lm n lm n lm jk lm jkA dx x A x dx A x dx A dx x           
Now for taking j k m
A dx x common , changes to be made in I , II & III terms { I =
lj , nk , II = lj, mk, & III = km & mk }
Then the Above Equation becomes
i
SRA - i
SQA = , ,
i j k m i j m k i l k m i l j k m
jm k jk m lk jm lm jkA dx x A x dx dx x A dx x           
= , ,[ ]i i i l i l j k m
jm k jk m lk jm lm jk A dx x        
= .
i j k m
j mkR A dx x
Where . , ,
i i i i l i l
j mk jm k jk m lk jm lm jkR          
Since j
A , k
dx and m
x are arbitrary vectors , it follows from the Quotient law
that .
i
j mkR is a tensor of rank 4. It is known as Riemann – Christoffel Curvature
Tensor . It is independent of the vector i
A and depends only on the metric
tensor and its I and II derivatives
RIEMANN –CHRISTOFFEL CURVATURE Tensor Identically vanishes at Euclidean
Space