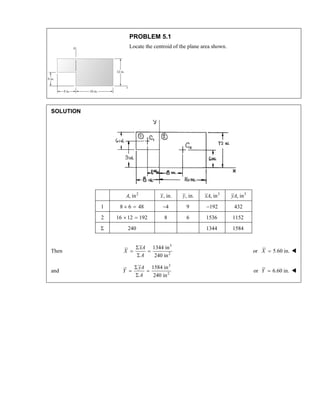
Vector mechanics for engineers statics 7th chapter 5

PROBLEM 5.1
Locate the centroid of the plane area shown.

SOLUTION

A, in 2 x , in. y , in. xA, in 3 yA, in 3
1 8 × 6 = 48 −4 9 −192 432
2 16 × 12 = 192 8 6 1536 1152
Σ 240 1344 1584

Σ xA 1344 in 3
Then X = = or X = 5.60 in.
ΣA 240 in 2

Σ yA 1584 in 3
and Y = = or Y = 6.60 in.
ΣA 240 in 2

PROBLEM 5.2

SOLUTION

A, mm 2 x , mm y , mm xA, mm3 yA, mm3
1
1 × 60 × 75 = 2250 40 25 90 000 56 250
2

2 105 × 75 = 7875 112.5 37.5 885 900 295 300

Σ 10 125 975 900 351 600

ΣxA 975 900 mm3
Then X = = or X = 96.4 mm
ΣA 10 125 mm 2
Σ yA 351 600 mm3
and Y = = or Y = 34.7 mm
ΣA 10 125 mm 2

PROBLEM 5.3

SOLUTION

For the area as a whole, it can be concluded by observation that
2
Y = ( 24 in.) or Y = 16.00 in.
3

A, in 2 x , in. xA, in 3
1 2
1 × 24 × 10 = 120 (10 ) = 6.667 800
2 3
1 1
2 × 24 × 16 = 192 10 + (16 ) = 15.333 2944
2 3

Σ 312 3744

Σ xA 3744 in 3
Then X = = or X = 12.00 in.
ΣA 312 in 2

PROBLEM 5.4

SOLUTION

1 21 × 22 = 462 1.5 11 693 5082
1
2 − ( 6 )( 9 ) = −27 −6 2 162 −54
2
1
3 − ( 6 )(12 ) = −36 8 2 −288 −72
2
Σ 399 567 4956

Σ xA 567 mm3
Then X = = or X = 1.421 mm
ΣA 399 mm 2
Σ yA 4956 mm3
and Y = = or Y = 12.42 mm
ΣA 399 mm 2

PROBLEM 5.5

SOLUTION

1 120 × 200 = 24 000 60 120 1 440 000 2 880 000

π ( 60 )
2
2 − = −5654.9 94.5 120 −534 600 −678 600
2
Σ 18 345 905 400 2 201 400

Σ xA 905 400 mm3
ΣA 18 345 mm 2

Σ yA 2 201 400 mm3
and Y = = or Y = 93.8 mm
ΣA 18 345 mm 2

PROBLEM 5.6

SOLUTION

A, in 2 x , in. y , in. x A, in 3 y A, in 3
π (9)
2 −4 ( 9 )
1 = 63.617 = −3.8917 3.8917 −243 243
4 ( 3π )
1
2 (15)( 9 ) = 67.5 5 3 337.5 202.5
2

Σ 131.1 94.5 445.5

Σ xA 94.5 in 3
Then X = = or X = 0.721 in.
ΣA 131.1 in 2

Σ yA 445.5 in 3
and Y = = or Y = 3.40 in.
ΣA 131.1 in 2

PROBLEM 5.7

SOLUTION

First note that symmetry implies X = Y

A, mm 2 x , mm xA, mm3

1 40 × 40 = 1600 20 32 000
π (40) 2
2 − = −1257 16.98 −21 330
4

Σ 343 10 667

Σ xA 10 667 mm3
ΣA 343 mm 2

and Y = X = 31.1 mm

PROBLEM 5.8

SOLUTION

First note that symmetry implies X =0

A, in 2 y , in. yA, in 3
π ( 4)
2
1 − = −25.13 1.6977 −42.67
2

π ( 6)
2
2 = 56.55 2.546 144
2

Σ 31.42 101.33

Σ yA 101.33 in 3
Then Y = = or Y = 3.23 in.
ΣA 31.42 in 2

PROBLEM 5.9
For the area of Problem 5.8, determine the ratio r2 /r1 so that y = 3r1/4.

SOLUTION

A y yA
π 4r1 2
1 − r12 − r13
2 3π 3
π 4r2 2 3
2 r22 r2
2 3π 3
π
Σ
2
(r 2
2
− r12 ) 3
(
2 3
r2 − r13 )

Then Y ΣA = Σy A

π 2
or
3
4
r1 ×
2
(
r2 − r12 =
2 3
3
r2 − r13) ( )
9π  r2    r 3
2
  − 1 =  2  − 1
16  r1    r1 
 
r2
Let p=
r1

9π
16
[ ( p + 1)( p − 1)] = ( p − 1)( p 2 + p + 1)
or 16 p 2 + (16 − 9π) p + (16 − 9π) = 0

PROBLEM 5.9 CONTINUED

−(16 − 9π) ± (16 − 9π) 2 − 4(16)(16 − 9π)
Then p=
2(16)

or p = −0.5726 p = 1.3397
r2
Taking the positive root = 1.340
r1

PROBLEM 5.10
Show that as r1 approaches r2 , the location of the centroid approaches that
of a circular arc of radius ( r1 + r2 ) / 2.

SOLUTION

First, determine the location of the centroid.

2 sin 2 − α (π )
From Fig. 5.8A: y2 = r2 π A2 = ( π2 − α ) r22
3 2
−α ( )
2 cos α
= r2 π
3 2(−α )
2 cos α
Similarly y1 = r1 A1 = ( π2 − α ) r12
3 π −α
2( )
2 cosα  π 2 cosα  π
Then Σ yA = r2 π (
− α r22  − r1 π ) − α r12  ( )
3 (
2
−α  2 )  3
2
−α  2  ( )
=
2 3
3
(r2 − r13 cosα)
π  π 
and Σ A =  − α  r22 −  − α  r12
2  2 
π  2
=  − α  r2 − r1
2 
2
( )
Now Y Σ A = Σ yA
 π  2 3

Y  − α  r22 − r12  =
 2 
( 3
)
r2 − r13 cos α ( )
2 r23 − r13 cos α
Y =
3 r22 − r12 π − α
2


1
Using Figure 5.8B, Y of an arc of radius ( r1 + r2 ) is
2

Y =
1 sin − α
( r1 + r2 ) π 2
(π )
2 (
2
−α )
1 cos α
= (r1 + r2 ) π (1)
2 ( 2
−α )
Now
r23 − r13
=
(
( r2 − r1 ) r22 + r1 r2 + r12 )
r22 − r12 ( r2 − r1 )( r2 + r1 )
r + r1 r2 + r12
2
= 2
r2 + r1
Let r2 = r + ∆
r1 = r − ∆
1
Then r = ( r1 + r2 )
2

and
r23 − r13
=
( r + ∆ ) + ( r + ∆ )( r − ∆ ) + ( r − ∆ )
2 2

2
r2 − r1 2
(r + ∆) + (r − ∆)
3r 2 + ∆ 2
=
2r
In the limit as ∆ → 0 (i.e., r1 = r2 ), then
r23 − r13 3
2 2
= r
r2 − r1 2
3 1
= × (r1 + r2 )
2 2
2 3 cos α 1 cos α
so that Y = × ( r1 + r2 ) π or Y = ( r1 + r2 ) π
3 4 2
−α 2 2
−α
Which agrees with Eq. (1).

PROBLEM 5.11

SOLUTION

First note that symmetry implies X =0

r2 = 2 2 in., α = 45°

y2 =
′
2r sin α 2 2 2 sin
=
( ) ( ) = 1.6977 in.
π
4
3α 3 π
4 ( )
A, in 2 y , in. y A, in 3
1
1 ( 4)(3) = 6 1 6
2
π
(2 2 )
2
2 = 6.283 2 − y ′ = 0.3024 1.8997
4
1
3 − ( 4)( 2) = −4 0.6667 −2.667
2

Σ 8.283 5.2330

Then Y Σ A = Σ yA

( )
Y 8.283 in 2 = 5.2330 in 3 or Y = 0.632 in.

PROBLEM 5.12

SOLUTION


1 (40)(90) = 3600 −15 20 −54 000 72 000
π ( 40)( 60)
2 = 2121 10 −15 6750 −10 125
4
1
3 (30)( 45) = 675 −25.47 −19.099 −54 000 −40 500
2

Σ 6396 −101 250 21 375

Then XA = Σ xA

( )
X 6396 mm 2 = −101 250 mm3 or X = −15.83 mm

and YA = Σ yA

( )
Y 6396 mm 2 = 21 375 mm3 or Y = 3.34 mm

PROBLEM 5.13

SOLUTION

2
1 ( 40)(80) = 2133 48 15 102 400 32 000
3
1
2 − ( 40)(80) = −1600 53.33 13.333 −85 330 −21 330
2

Σ 533.3 17 067 10 667

Then X Σ A = Σ XA

( )
X 533.3 mm 2 = 17 067 mm3 or X = 32.0 mm

and Y Σ A = Σ yA

( )
Y 533.3 mm 2 = 10 667 mm3 or Y = 20.0 mm

PROBLEM 5.14

SOLUTION

2
1 (150 )( 240 ) = 24 000 56.25 96 1 350 000 2 304 000
3
1
2 − (150)(120) = −9000 50 40 −450 000 −360 000
2

Σ 15 000 900 000 1 944 000

Then X Σ A = Σ xA

( )
X 15 000 mm 2 = 900 000 mm3 or X = 60.0 mm

and Y Σ A = Σ yA

( )
Y 15 000 mm 2 = 1 944 000 or Y = 129.6 mm

PROBLEM 5.15

SOLUTION

1
1 (10)(15) = 50 4.5 7.5 225 375
3
π
2 (15)2 = 176.71 6.366 16.366 1125 2892
4

Σ 226.71 1350 3267

Then X Σ A = Σx A

( )
X 226.71 in 2 = 1350 in 3 or X = 5.95 in.

and Y ΣA = Σy A

( )
Y 226.71 in 2 = 3267 in 3 or Y = 14.41 in.

PROBLEM 5.16

SOLUTION

2
1 (8)(8) = 42.67 3 2.8 128 119.47
3
2
2 − ( 4)( 2) = −5.333 1.5 −0.8 −8 4.267
3

Σ 37.33 120 123.73

Then X Σ A = Σx A

( )
X 37.33 in 2 = 120 in 3 or X = 3.21 in.

and Y ΣA = Σy A

( )
Y 37.33 in 2 = 123.73 in 3 or Y = 3.31 in.

PROBLEM 5.17
The horizontal x axis is drawn through the centroid C of the area shown
and divides the area into two component areas A1 and A2. Determine
the first moment of each component area with respect to the x axis, and
explain the results obtained.

SOLUTION

Note that Qx = Σ yA

Then (Qx )1 =  5

3
1
2

m  × 6 × 5 m 2

or ( Qx )1 = 25.0 × 103 mm3

 2  1   1  1 
and ( Qx )2 =  − × 2.5 m  × 9 × 2.5  m 2 +  − × 2.5 m  × 6 × 2.5  m 2
 3  2   3  2 

or ( Qx ) 2 = −25.0 × 103 mm3

Now Qx = ( Qx )1 + ( Qx ) 2 = 0

This result is expected since x is a centroidal axis ( thus y = 0 )

and Qx = Σ y A = Y Σ A (y = 0 ⇒ Qx = 0 )

PROBLEM 5.18
The horizontal x axis is drawn through the centroid C of the area shown
and divides the area into two component areas A1 and A2. Determine
the first moment of each component area with respect to the x axis, and
explain the results obtained.

SOLUTION

First, locate the position y of the figure.

A, mm 2 y , mm yA, mm3
1 160 × 300 = 48 000 150 7 200 000

2 −150 × 80 = −16 000 160 −2 560 000

Σ 32 000 4 640 000

Then Y ΣA = Σy A

( )
Y 32 000 mm 2 = 4 640 000 mm3

or Y = 145.0 mm


A I: Q I = Σ yA
155 115
= (160 × 155) +  − ( 80 × 115) 
2 2  
6 3
= 1.393 × 10 mm
A II : Q II = Σ yA
145  85 
=− (160 × 145) −  − 2 ( 80 × 85 ) 
2  
= −1.393 × 106 mm3
∴ ( Qarea ) x = QI + QII = 0

Which is expected since Qx = Σ yA = yA and y = 0 ,
since x is a centroidal axis.

PROBLEM 5.19
The first moment of the shaded area with respect to the x axis is denoted
by Qx . (a) Express Qx in terms of r and θ . (b) For what value of θ is
Qx maximum, and what is the maximum value?

SOLUTION

(a) With Qx = Σ yA and using Fig. 5.8 A,

(
 2 r sin π − θ )
Qx =  3 π 2
 −θ 
(
2  ) ( 3 r sin θ )  1 × 2r cos θ × r sin θ 
 r 2 π − θ  − 2

2


 2 
2
(
= r 3 cos θ − cos θ sin 2 θ
3
)
2 3
or Qx = r cos3 θ
3
(b) By observation, Qx is maximum when θ =0
2 3
and then Qx = r
3

PROBLEM 5.20
A composite beam is constructed by bolting four plates to four
2 × 2 × 3/8-in. angles as shown. The bolts are equally spaced along the
beam, and the beam supports a vertical load. As proved in mechanics of
materials, the shearing forces exerted on the bolts at A and B are
proportional to the first moments with respect to the centroidal x axis of
the red shaded areas shown, respectively, in parts a and b of the figure.
Knowing that the force exerted on the bolt at A is 70 lb, determine the
force exerted on the bolt at B.

SOLUTION
From the problem statement: F ∝ Qx
FA FB
so that =
(Qx ) A (Qx ) B
(Qx ) B
and FB = F
(Qx ) A A
Now Qx = ∑ yA
 0.375 
So ( Qx ) A =  7.5 in. + in.  10 in. × ( 0.375 in.)  = 28.82 in 3
 
 2 

 0.375 
and ( Qx )B = ( Qx ) A + 2  7.5 in. − in.  (1.625 in.)( 0.375 in.) 
 
 2 
+ 2 ( 7.5 in. − 1 in.) ( 2 in.)( 0.375 in.) 
 

= 28.82 in 3 + 8.921 in 3 + 9.75 in 3

= 47.49 in 3

47.49 in 3
Then FB = ( 70 lb) = 115.3 lb
28.82 in 3

PROBLEM 5.21
A thin, homogeneous wire is bent to form the perimeter of the figure
indicated. Locate the center of gravity of the wire figure thus formed.

SOLUTION
First note that because the wire is homogeneous, its center of gravity will
coincide with the centroid of the corresponding line.

L, in. x , in. y , in. xL, in 2 yL, in 2
1 16 8 0 128 0
2 12 16 6 102 72
3 24 4 12 96 288
4 6 −8 9 −48 54
5 8 −4 6 −32 48
6 6 0 3 0 18
Σ 72 336 480

Then X ΣL = Σ x L

X ( 72 in.) = 336 in 2 or X = 4.67 in.

and Y ΣL = Σ y L

Y (72 in.) = 480 in 2 or Y = 6.67 in.

PROBLEM 5.22

SOLUTION

First note that because the wire is homogeneous, its center of gravity will coincide with the centroid of the
corresponding line.

L, mm x , mm y , mm xL, mm 2 yL, mm 2
1 165 82.5 0 13 612 0
2 75 165 37.5 12 375 2812
3 105 112.5 75 11 812 7875
4 602 + 752 = 96.05 30 37.5 2881 3602
Σ 441.05 40 680 14 289

Then X ΣL = Σx L

X (441.05 mm) = 40 680 mm 2 or X = 92.2 mm

and Y ΣL = Σ y L

Y (441.05 mm) = 14 289 mm 2 Y = 32.4 mm

PROBLEM 5.23

SOLUTION

First note that because the wire is homogeneous, its center of gravity will coincide with the centroid of the
corresponding line.

L, mm x , mm y , mm xL, mm 2 yL, mm 2

1 122 + 62 = 13.416 6 3 80.50 40.25
2 16 12 14 192 224
3 21 1.5 22 31.50 462
4 16 −9 14 −144 224

5 62 + 92 = 10.817 −4.5 3 −48.67 32.45
Σ 77.233 111.32 982.7

Then X ΣL = Σx L

X (77.233 mm) = 111.32 mm 2 or X = 1.441 mm

and Y ΣL = Σ y L

Y (77.233 mm) = 982.7 mm 2 or Y = 12.72 mm

PROBLEM 5.24

SOLUTION
First note that because the wire is homogeneous, its center of gravity will
coincide with the centroid of the corresponding line.
By symmetry X =0

L, in. y , in. yL, in 2
1 2 0 0

π ( 6) 2 ( 6)
2 = 3.820 72
π
3 2 0 0

4 π ( 4) 2 ( 4)
= 2.546 32
π
Σ 35.416 104

Then Y ΣL = Σ y L

Y (35.416 in.) = 104 in 2 or Y = 2.94 in.

PROBLEM 5.25
A 750 = g uniform steel rod is bent into a circular arc of radius 500 mm
as shown. The rod is supported by a pin at A and the cord BC. Determine
(a) the tension in the cord, (b) the reaction at A.

SOLUTION
First note, from Figure 5.8B: X =
( 0.5 m ) sin 30°
π/6

1.5
= m
π
Then W = mg
(
= ( 0.75 kg ) 9.81 m/s 2 )
= 7.358 N
Also note that ∆ ABD is an equilateral triangle.
Equilibrium then requires

(a) ΣM A = 0:
  1.5  
0.5 m −  m  cos 30° ( 7.358 N ) − ( 0.5 m ) sin 60° TBC = 0
 
  π  
or TBC = 1.4698 N or TBC = 1.470 N

(b) ΣFx = 0: Ax + (1.4698 N ) cos 60° = 0

or Ax = −0.7349 N

ΣFy = 0: Ay − 7.358 N + (1.4698 N ) sin 60° = 0

or Ay = 6.085 N thus A = 6.13 N 83.1°

PROBLEM 5.26
The homogeneous wire ABCD is bent as shown and is supported by a pin
at B. Knowing that l = 8 in., determine the angle θ for which portion
BC of the wire is horizontal.

SOLUTION
First note that for equilibrium, the center of gravity of the wire must lie
on a vertical line through B. Further, because the wire is homogeneous,
its center of gravity will coincide with the centroid of the corresponding
line.
Thus ΣM B = 0, which implies that x = 0 or ΣxL = 0

Hence

(π × 6 in.) + 
2(6 in.) 8 in. 
−   ( 8 in.)
π  2 

 6 in. 
+  8 in. − cosθ  ( 6 in.) = 0
 2 

4
Then cosθ = or θ = 63.6°
9

PROBLEM 5.27
The homogeneous wire ABCD is bent as shown and is supported by a pin
at B. Knowing that θ = 30°, determine the length l for which portion CD
of the wire is horizontal.

SOLUTION
First note that for equilibrium, the center of gravity of the wire must lie on
a vertical line through B. Further, because the wire is homogeneous, its
center of gravity will coincide with the centroid of the corresponding line.
Thus ΣM B = 0, which implies that x = 0 or Σxi Li = 0

 2 ( 6 in.) 
Hence − cos 30° + ( 6 in.) sin 30°  (π × 6 in.)
 π 

 ( l in.) 
+ cos 30° ( l in.)
 2 

 6 in. 
+ ( l in.) cos 30° − ( 6 in.) = 0
 2  

or l 2 + 12.0l − 316.16 = 0

with roots l1 = 12.77 and −24.77.
Taking the positive root

l = 12.77 in.

PROBLEM 5.28
The homogeneous wire ABCD is bent as shown and is attached to a hinge
at C. Determine the length L for which the portion BCD of the wire is
horizontal.

SOLUTION
First note that for equilibrium, the center of gravity of the wire must lie on
a vertical line through C. Further, because the wire is homogeneous, its
center of gravity will coincide with the centroid of the corresponding line.
Thus ΣM C = 0, which implies that x = 0

or Σ xi Li = 0

L
Hence ( L ) + ( −4 in.)(8 in.) + ( −4 in.)(10 in.) = 0
2

or L2 = 144 in 2 or L = 12.00 in.

PROBLEM 5.29
Determine the distance h so that the centroid of the shaded area is as close
to line BB′ as possible when (a) k = 0.2, (b) k = 0.6.

SOLUTION

ΣyA
Then y =
ΣA

a  (a + h) 
( ab ) −    kb ( a − h ) 
 
2  2 
or y =
ba − kb ( a − h )

1 a (1 − k ) + kh
2 2
=
2 a(1 − k ) + kh

h
Let c =1− k and ζ =
a

a c + kζ 2
Then y = (1)
2 c + kζ
Now find a value of ζ (or h) for which y is minimum:

(
a 2kζ ( c + kζ ) − k c + kζ
2
)
dy
dζ
=
2 ( c + kζ ) 2
=0 or ( )
2ζ ( c + kζ ) − c + kζ 2 = 0 (2)


Expanding (2) 2cζ + 2ζ 2
− c − kζ 2
=0 or kζ 2
+ 2cζ − c = 0

−2c ± ( 2c) 2 − 4 ( k ) ( c )
Then ζ=
2k
Taking the positive root, since h > 0 (hence ζ > 0 )

−2 (1 − k ) + 4 (1 − k ) + 4k (1 − k )
2
h=a
2k

−2 (1 − 0.2 ) + 4 (1 − 0.2 ) + 4 ( 0.2 )(1 − 0.2 )
2
(a) k = 0.2: h=a or h = 0.472a
2 ( 0.2 )

−2 (1 − 0.6 ) + 4 (1 − 0.6 ) + 4 ( 0.6 )(1 − 0.6 )
2
(b) k = 0.6: h=a or h = 0.387a
2 ( 0.6 )

PROBLEM 5.30
Show when the distance h is selected to minimize the distance y from
line BB′ to the centroid of the shaded area that y = h.

SOLUTION

From Problem 5.29, note that Eq. (2) yields the value of ζ that minimizes h.

Then from Eq. (2)

c + kζ 2
We see 2ζ = (3)
c + kζ

Then, replacing the right-hand side of (1) by 2ζ , from Eq. (3)

a
We obtain y = ( 2ζ)
2

h
But ζ=
a

So y =h Q.E.D.

PROBLEM 5.31
Determine by direct integration the centroid of the area shown. Express
your answer in terms of a and h.

SOLUTION
h
For the element of area (EL) shown y = x
a
and dA = ( h − y ) dx
 x
= h 1 −  dx
 a
Then xEL = x
1
yEL = (h + y)
2
h x
= 1 + 
2 a
a
a  x  x2  1
Then area A = ∫ dA = ∫ h 1
0 
−  dx = h  x −  = 2 ah
 a  2a 
0

a
a   x   x 2 x3  1 2
and ∫ xEL dA = ∫x h 1
0  
−  dx  = h 
a   2 − 3a  = 6 a h

   0
a h x   x   h2 a  x2 
∫ yEL dA = ∫0 
2
1 +   h  1 −  dx  =
a   a  2 0
1 − 2 dx
∫  a 


a
h2  x3  1 2
=  x− 2 = ah
2  3a  3
0

Hence xA = ∫ xEL dA
1  1
x  ah  = a 2h
2  6
1
x = a
3
yA = ∫ yEL dA
1  1
y  ah  = ah 2
2  3
2
y = h
3

PROBLEM 5.32

SOLUTION
For the element (EL) shown
h
At x = a, y = h : h = ka3 or k =
a3
a 1/3
Then x= y
h1/3
Now dA = xdy
a 1/3
= y dy
h1/3
1 1 a 1/3
xEL = x= y , yEL = y
2 2 h1/ 3
h
Then A = ∫ dA =∫0
h

h
a 1/3
1/3
y dy =
3 a
4 h1/3
( )
y 4/3
0
=
3
4
ah

h
h 1 a 1/3  a 1/3  1 a  3 5/3  3 2
and ∫ xEL dA = ∫0 y  1/3 y dy  =  y  = a h
2 h1/3 h  2 h 2/3  5 0 10
h
h  a 1/3  a  3 7/3  3 2
∫ yEL dA = ∫0 y  h1/3 y dy  = h1/3  7 y  = 7 ah
   0

3  3 2 2
Hence xA = ∫ xEL dA : x  ah  = a h x = a
 4  10 5
3  3 4
yA = ∫ yEL dA: y  ah  = ah 2 y = h
4  7 7

PROBLEM 5.33

SOLUTION
h
At x = a, y = h: h = k1a3 or k1 =
a3
a
a = k 2 h3 or k2 =
h3
Hence, on line 1
h 3
y = x
a3
and on line 2
h 1/3
y = x
a1/3
Then
 h h  1  h 1/3 h 3
dA =  1/3 x1/3 − 3 x3  dx and yEL =  1/3 x + 3 x 
a a  2 a a 
a
a h h   3 1  1
∴ A = ∫ dA = ∫0  1/3 x1/3 − 3 x3  dx = h  1/3 x 4/3 − 3 x 4  = ah
 a a   4a 4a 0 2
a
a  h 1/3 h   3 1  8 2
∫ xELdA = ∫ x
0  1/3
x − 3 x3  dx = h  1/3 x7/3 − 3 x5  = a h
a a   7a 5a  0 35

a 
1 h 1/3 h 3  h
1/3 3 h
∫ yEL dA = ∫0 2  a1/3 x + a3 x  a1/3 x − a3 x  dx
  
a
h 2 a  x 2/3 x 6  h 2  3 x5/3 1 x 6  8 2
=  2/3 − 6  dx =
∫0  a   5 a5/3 − 7 a 6  = 35 ah
 
2  a  2  0

 ah  8 2 16
From xA = ∫ xEL dA: x   = a h or x = a
 2  35 35

 ah  8 2 16
and yA = ∫ yEL dA: y   = ah or y = h
 2  35 35

PROBLEM 5.34
Determine by direct integration the centroid of the area shown.

SOLUTION
First note that symmetry implies x =0
2r
yEL = (Figure 5.8B)
π
dA = π rd r
r2
 r2  π 2
Then A = ∫ dA = ∫
r2
r1
π rd r =π  =
 2 2
r2 − r12 ( )
  r1

r2
and
r
∫ yEL dA = ∫r1
2 2r
π
1
3  r 3

( π rd r ) = 2  r 3  = r23 − r13
2
( )
1

π
So (
 2 3
yA = ∫ yEL dA: y  r22 − r12  =
2  3
)
r2 − r13 ( )
4 r23 − r13
or y =
3π r22 − r12

PROBLEM 5.35

SOLUTION
First note that symmetry implies x =0
y = R cos θ, x = R sin θ
dx = R cos θ d θ

dA = ydx = R 2 cos 2θ dθ
Hence
α
α  θ sin 2θ  1 2
A = ∫ dA = 2∫0 R 2 cos 2 θ dθ = 2 R 2  +  = R ( 2α sin 2α )
 2 4 0 2
α
α R
( 31
) 2 
∫ yEL dA = 2∫0 2 cosθ R cos θ dθ = R  3 cos θ sin θ + 3 sin θ 
2 2

2
0
R3
=
3
(
cos 2 α sin α + 2sin α )
R3
3
(
cos 2 α sin α + 2sin α )
But yA = ∫ yEL dA so y =
R2
( 2α + sin 2α )
2

or
2
y = R sin α
(cos 2 α + 2 )
3 ( 2α + sin 2α )
2 3 − sin 2 α
Alternatively, y = R sin α
3 2α + sin 2α

PROBLEM 5.36

SOLUTION
b 2
y = a − x2
a
and dA = ( b − y ) dx

=
b
a( )
a − a 2 − x 2 dx

a+ a −x )
2a (
1 b
xEL = x; y = ( y + b ) =
EL
2 2
2

A = ∫ dA = ∫ ( a − a − x ) dx
b a 2 2
Then 0
a

To integrate, let x = a sin θ : a 2 − x 2 = a cosθ , dx = a cosθ dθ

π /2 b
Then A = ∫0 ( a − a cosθ )( a cosθ dθ )
a
π /2
b θ 2θ    π
=  a 2 sin θ − a 2  + sin  = ab  1 − 
a 2 4  0  4
π /2
a b
(  b  a
) 1
and ∫ xEL dA = ∫0 x  a − a 2 − x 2 dx  =  x 2 + a 2 − x 2
 a  a  2 3
( )
3/2  

 0
1 3
= ab
6
a b
(
2 2 b 2
) (
2 
∫ yEL dA = ∫0 2a a + a − x  a a − a − x dx 
 
)
a
b2 a 2 b 2  x3 
=
2a 2 ∫0
x dx =( )  
2a 2  3 
=
1 2
6
ab
  0

  π  1 2a
xA = ∫ xEL dA: x  ab 1 −   = a 2b or x =
  4  6 3 ( 4 − π)

  π  1 2b
yA = ∫ yEL dA: y  ab 1 −   = ab 2 or y =
  4  6 3 ( 4 − π)

PROBLEM 5.37
your answer in terms of a and b.

SOLUTION
For the element (EL) shown on line 1 at
b
x = a, b = k2a 2 or k2 =
a2
b 2
∴ y = x
a2
−2b
On line 2 at x = a, −2b = k1a3 or k2 =
a3
−2b 3
∴ y = x
a3
 b 2b 
dA =  2 x 2 + 3 x3  dx
a a 
a
b 
a 2 x3  b  x3 2 x 4 
Then A = ∫ dA = ∫ 2  x 2 + dx = 2  +
0
a  x   a  3 4a   0

 1 1 5
= ab  +  = ab
 3 2 6
a
a  b 2 2b 3  b  x 4 2 x5  2 1 2
and ∫ xEL dA = ∫ x
0  2
x + 3 x  dx = 2 
 4 + 5a  = a b  4 + 5 

a a  a  0  
13 2
= ab
20
a1 b 2 2b 3   b 2 2b 3  
∫ yEL dA = ∫0 2  a 2 x − a3 x   a 2 x + a3 x  dx 
    
a
1  b
2 2
a   2b  b 2  x5 2 7
= ∫  2 x 2  −  3 x 3 
0
 dx =  5 − 7a 2 x 
4 
2  a
  a  
 2a  0
 1 2 13
= b 2a5  −  = − ab 2
 10 7  70
 5  13 2 39
Then xA = ∫ xEL dA: x  ab  = ab or x = a
 6  20 50
 5  13 2 39
yA = ∫ yEL dA: y  ab  − ab or y = − b
 6  70 175

PROBLEM 5.38

SOLUTION
At x = 0, y = b

b
b = k (0 − a)
2
or k =
a2
b
Then y = ( x − a )2
a2
y b
2(
x − a)
2
Now xEL = x, yEL = =
2 2a
b
and dA = ydx = ( x − a )2 dx
a2
b b a 1
2(
x − a ) dx = 2 ( x − a )  = ab
a 2 3
Then A = ∫ dA = ∫0  0 3
a 3a  

∫ xEL dA = ∫0 x  a 2 ( x − a ) dx  = a 2 ∫0 ( x − 2ax + a x )dx
a  b 2  a 3b 2 2
and
 
b  x4 2 3 a2 2  1 2
=  4 − 3 ax + 2 x  = 12 a b
2 
a  
a
b 2 b   b2 1 5
∫ yEL dA = ∫0 2a 2 ( x − a )  a 2 ( x − a ) dx  = 2a 4  5 ( x − a ) 
a 2

   0
1 2
= ab
10
1  1 2 1
Hence xA = ∫ xEL dA: x  ab  = ab x = a
 3  12 4

1  1 2 3
yA = ∫ yEL dA: y  ab  = ab y = b
 3  10 10

PROBLEM 5.39

SOLUTION
Have xEL = x
1 a x x2 
yEL = y = 1 − + 2 
2 2
 L L 
 x x2 
dA = ydx = a 1 − + 2  dx

 L L 
2L
2L  x x2   x2 x3  8
Then A = ∫ dA = ∫ 0
− + 2  dx = a  x −
a 1 + 2  = aL
 L L   2 L 3L 0 3

2L
2L   x x2    x2 x3 x4 
and ∫ xEL dA = ∫0 x  a 1 − L + L2  dx  = a  2 − 3L + 4L2 
 
 
     0
10 2
= aL
3
2L a x x2    x x2  
∫ yEL dA = ∫0 
2
1 − + 2   a  1 − + 2  dx 
L L   L L  
    
a 2 EL  x x2 x3 x 4 
= ∫0 1 − 2 L + 3 L2 − 2 L3 + L4  dx
 
2  
2L
a2  x 2 x3 x4 x5  11
= x − + 2 − 3 + 4  = a2L
2  L L 2L 5L  0 5

 8  10 2 5
Hence xA = ∫ xEL dA: x  aL  = aL x = L
3  3 4

 1  11 33
yA = ∫ yEL dA: y  a  = a 2 y = a
8  5 40

PROBLEM 5.40

SOLUTION
2b
For y1 at x = a, y = 2b 2b = ka 2 or k =
a2
2b 2
Then y1 = x
a2

( x + 2b) = b  2 − 
b x
By observation y2 = −  
a  a

Now xEL = x
and for 0 ≤ x ≤ a :
1 b 2b 2
yEL = y1 = 2 x 2 and dA = y1dx = x dx
2 a a2
For a ≤ x ≤ 2a :

1 b x  x
yEL = y2 =  2 −  and dA = y2dx = b  2 −  dx
2 2 a  a

a 2b 2 2a  x
Then A = ∫ dA = ∫0 2
x dx + ∫a b  2 − dx
a  a
a 2a
2b  x3   a x 
2
7
= 2   + b  −  2 −   = ab
a  3 0  2
 a 0 6

a  2b 2  2a   x 
and ∫ xEL dA = ∫0 x  a 2 x dx  + ∫a x b  2 − a  dx 
     
a 2a
2b  x 4   2 x3 
=   + b x − 
a2  4 0  3a  0

=
1 2
2 
 {
a b + b  ( 2a ) − ( a )  +
2 2

1  2
 ( )
3
 3a  2a − ( a )  

7 2
= ab
6


a b 2  2b 2  2a b  x  x 
∫ yEL dA = ∫0 a 2 x  a 2 x dx  + ∫0 2  2 − a  b  2 − a  dx 
      
a 2a
b2  a  x 
3
2b 2  x5 
= 4   + −  2 −  
a  5 0 2  3
 a a
17 2
= ab
30

7  7
Hence xA = ∫ xEL dA: x  ab  = a 2b x =a
6  6

 7  17 2 17
yA = ∫ yEL dA: y  ab  = ab y = b
 6  30 35

PROBLEM 5.41

SOLUTION
a
For y2 at x = a, y = b : a = kb 2 or k =
b2
b 1/2
Then y2 = x
a
Now xEL = x
a y b x1/2 x1/2
and for 0≤ x≤ : yEL = 2 = , dA = y2dx = b dx
2 2 2 a a

a 1 b  x 1 x1/2 
For ≤ x ≤ a : yEL = ( y1 + y2 ) =  − + 
2 2 2a 2
 a 

 x1/2 x 1 
dA = ( y2 − y1 ) dx = b  − +  dx
 a a 2

a/2 x1/2 a  x1/2 x 1 
Then A = ∫ dA = ∫0 b dx + ∫a/2 b 
 a − a + 2  dx

a  
a/2 a
b  2 3/2   2 x3/2 x2 1 
= 3 x  + b − + x
a  0 3 a 2a 2  a/2

2 b  a  a 
3/2 3/2
  + ( a ) −   
3/2
=
3 a  2 
 2  
 1  
a  1
2
 a  

( )
+ b  −  a −    + ( a ) −    
2

 2a 
  2  2
  2  

13
= ab
24


a/2  x
1/2  a   x1/2 x 1  
and ∫ xEL dA = ∫0 x  b
 dx  + ∫a/2 x b  − +  dx
 a   

  a a 2 


a/2 a
b  2 5/2   2 x5/2 x3 x 4 
= x  + b − + 
a 5
 0  5 a 3a 4  a/2

2 b  a  a 
5/2 5/2
  + ( a ) −   
5/2
=
5 a  2 
 2  
 1  3  a 3  1  2  a  2  
 
+ b  − ( a ) −    +  ( a ) −    
 3a 
  2  4
   2  


71 2
= ab
240
a/2 b x1/2  x1/2 
∫ yEL dA = ∫0 2 b dx 
a a 

a b x 1 x1/2    x1/2 x 1  
+ ∫a/2  − +  b  − +  dx 
2 a 2
 a   a a 2  
   
a
b 2  x 2 1  x 1  
a/2 3
b2  1 2 
= x +  −  −  
2a  2  0
  
2  2a 3a  a 2    
  a/2

b  a 
2 2 3
a b2  a 1 
  + ( a ) −  
2
= −  − 
4a   2 
 2  6a  2 2 

11 2
= ab
48
 13  71 2 17
Hence xA = ∫ xEL dA: x  ab  = ab x = a = 0.546a
 24  240 130

 13  11 2 11
yA = ∫ yEL dA: y  ab  = ab y = b = 0.423b
 24  48 26

PROBLEM 5.42
A homogeneous wire is bent into the shape shown. Determine by direct
integration the x coordinate of its centroid. Express your answer in terms
of a.

SOLUTION
First note that because the wire is homogeneous, its center of gravity
coincides with the centroid of the corresponding line
1
Have at x = a, y = a : a = ka 2 or k =
a
1 2 2
Thus y = x and dy = xdx
a a
2 2
 dy  2 
Then dL = 1 +   dx = 1 +  x  dx
 dx  a 
a
4 x 4x2 a 2 4x2 
a
∴ L = ∫ dL = ∫ 1 + 2 x 2 dx =  1 + 2 + ln  x + 1 + 2 
0
a 2 a 4 a a 
  0

=
a
2
a
(
5 + ln 2 + 5 = 1.4789a
4
)
a
a
 4x2   2  a2   4  
3/2

∫ xELdL = ∫ x 1 + 2 dx  =    1 + 2 x 2  
0 
a   3  8  a  
     0
a 2 3/2
=
12
( )
5 − 1 = 0.8484a 2

Then xL = ∫ xEL dL: x (1.4789a ) = 0.8484a 2 x = 0.574a

PROBLEM 5.43
integration the x coordinate of its centroid.

SOLUTION

Now xEL = r cos θ and dL = rd θ

7π /4 7π /4 3
Then L = ∫ dL = ∫π /4 rdθ = r [θ ]π /4 = πr
2
7π /4
and ∫ xELdL = ∫π /4 r cosθ ( rdθ )

7π /4  1 1 
= r 2 [sin θ ]π /4 = r 2  − − 2
 = −r 2
 2 2

3  2 2
Thus xL = ∫ xdL : x  π r  = −r 2 2 x =− r
2  3π

PROBLEM 5.44
integration the x coordinate of its centroid.

SOLUTION

Now xEL = a cos3 θ and dL = dx 2 + dy 2

Where x = a cos3 θ : dx = −3a cos 2 θ sin θ dθ

y = a sin 3 θ : dy = 3a sin 2 θ cosθ dθ
1/2

( ) + (3a sin θ cosθ dθ ) 
2 2
Then dL =  −3a cos 2 θ sin θ dθ 2

 

( )
1/2
= 3a cosθ sin θ cos 2 θ + sin 2 θ dθ
= 3a cosθ sin θ dθ
π /2
π /2 1 
∴ L = ∫ dL = ∫0 3a cosθ sin θ dθ = 3a  sin 2 θ 
 2 0
3
= a
2
π /2
∫ xEL dL = ∫0 a cos θ ( 3a cosθ sin θ dθ )
3
and
π /2
 1  3 2
= 3a 2  − cos5 θ  = a
 5 0 5

3  3 2
Hence xL = ∫ xEL dL : x  a  = a 2 x = a
2  5 5

Vector mechanics for engineers statics 7th chapter 5

Vector mechanics for engineers statics 7th chapter 5

Recomendados

Recomendados

Mais conteúdo relacionado

Mais procurados

Mais procurados (20)

Semelhante a Vector mechanics for engineers statics 7th chapter 5

Semelhante a Vector mechanics for engineers statics 7th chapter 5 (20)

Último

Último (20)

Vector mechanics for engineers statics 7th chapter 5