972 B3102005 Cullity Chapter 2
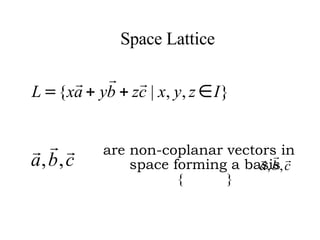
- 1. Space Lattice are non-coplanar vectors in space forming a basis { }
- 2. One dimensional lattice Two dimensional lattice
- 4. Lattice vectors and parameters
- 6. Miller indices for planes
- 7. Miller indices and plane spacing
- 8. Two-dimensional lattice showing that lines of lowest indices have the greatest spacing and greatest density of lattice points
- 10. Illustration of crystal lattices and corresponding reciprocal lattices for a cubic system
- 11. Illustration of crystal lattices and corresponding reciprocal lattices for a a hexagonal system
- 12. If then and perpendicular to (hkl) plane
- 13. If then and perpendicular to (hkl) plane Proof: H • ( a 1 /h- a 2 /k) = H • ( a 1 /h- a 3 /l)=0 a 1 /h• H /| H |=[1/h 0 0] • [hkl]*/| H |=1/| H |=d hkl H a 1 a 2 a 3
- 14. Symmetry (a) mirror plane (b)rotation (c)inversion (d)roto- inversion
- 16. Crystal system
- 17. The 14 Bravais lattices
- 18. The fourteen Bravais lattices Simple cubic lattices nitrogen - simple cubic copper - face centered cubic body centered cubic Cubic lattices a 1 = a 2 = a 3 α = β = γ = 90 o
- 19. Tetragonal lattices a1 = a2 ≠ a3 α = β = γ = 90 simple tetragonal Body centered Tetragonal
- 20. Orthorhombic lattices a1 ≠ a2 ≠ a3 α = β = γ = 90 simple orthorhombic Base centered orthorhombic Body centered orthorhombic Face centered orthorhombic
- 21. Monoclinic lattices a1 ≠ a2 ≠ a3 α = γ = 90 ≠ β (2nd setting) α = β = 90 ≠ γ (1st setting) Simple monoclinic Base centered monoclinic
- 22. Triclinic lattice a1 ≠ a2 ≠ a3 α ≠ β ≠ γ Simple triclinic
- 23. Hexagonal lattice a1 = a2 ≠ a3 α = β = 90 , γ = 120 lanthanum - hexagonal
- 24. Trigonal (Rhombohedral) lattice a1 = a2 = a3 α = β = γ ≠ 90 mercury - trigonal
- 25. Relation between rhombo-hedral and hexagonal lattices
- 26. Relation of tetragonal C lattice to tetragonal P lattice
- 27. Extension of lattice points through space by the unit cell vectors a, b, c
- 29. Primitive and non-primitive cells Face-centerd cubic point lattice referred to cubic and rhombo-hedral cells
- 30. All shaded planes in the cubic lattice shown are planes of the zone{001}
- 31. Zone axis [uvw] Zone plane (hkl) then hu+kv+wl=0 Two zone planes (h 1 k 1 l 1 ) and (h 2 k 2 l 2 ) then zone axis [uvw]=
- 32. Plane spacing
- 33. Indexing the hexagonal system
- 34. Indexing the hexagonal system
- 35. Crystal structure -Fe, Cr, Mo, V -Fe, Cu, Pb, Ni
- 36. Hexagonal close-packed Zn, Mg, Be, -Ti
- 37. FCC and HCP
- 38. -Uranium, base-centered orthorhombic (C-centered) y=0.105±0.005
- 43. AuBe: Simple cubic u = 0.100 w = 0.406
- 44. Structure of solid solution (a) Mo in Cr (substitutional) (b) C in -Fe (interstitial)
- 45. Atom sizes (d) and coordination
- 46. Change in coordination 12 8 12 6 12 4 size contraction, percent 3 3 12
- 47. A: Octahedral site, B: Tetrahedral site
- 48. Twin
- 49. (a) (b) FCC annealing (c) HCP deformation twins
- 50. Twin band in FCC lattice, Plane of main drawing is (1 ī 0)
- 51. Homework assignment Problem 2-6 Problem 2-8 Problem 2-9 Problem 2-10
- 52. Stereographic projection *Any plane passing the center of the reference sphere intersects the sphere in a trace called great circle * A plane can be represented by its great circle or pole, which is the intersection of its plane normal with the reference sphere
- 55. Pole on upper sphere can also be projected to the horizontal (equatorial) plane
- 56. Projections of the two ends of a line or plane normal on the equatorial plane are symmetrical with respect to the center O.
- 57. Projections of the two ends of a line or plane normal on the equatorial plane are symmetrical with respect to the center O. U L P P’ P P’ X O O
- 59. N S E W
- 60. The position of pole P can be defined by two angles and
- 61. The position of projection P’ can be obtained by r = R tan( /2)
- 62. The trace of each semi-great circle hinged along NS projects on WNES plane as a meridian
- 63. As the semi-great circle swings along NS, the end point of each radius draws on the upper sphere a curve which projects on WNES plane as a parallel
- 64. The weaving of meridians and parallels makes the Wulff net
- 65. Two projected poles can always be rotated along the net normal to a same meridian (not parallel) such that their intersecting angle can be counted from the net
- 66. P : a pole at ( 1 , 1 ) NMS : its trace
- 67. The projection of a plane trace and pole can be found from each other by rotating the projection along net normal to the following position
- 68. Zone circle and zone pole
- 69. If P2’ is the projection of a zone axis, then all poles of the corresponding zone planes lie on the trace of P2’
- 70. Rotation of a poles about NS axis by a fixed angle: the corresponding poles moving along a parallel *Pole A1 move to pole A2 *Pole B1 moves 40 ° to the net end then another 20 ° along the same parallel to B1’ corresponding to a movement on the lower half reference sphere, pole corresponding to B1’ on upper half sphere is B2
- 71. m: mirror plane F1: face 1 F2: face 2 N1: normal of F1 N2: normal of N2 N1, N2 lie on a plane which is 丄 to m
- 73. A plane not passing through the center of the reference sphere intersects the sphere on a small circle which also projects as a circle, but the center of the former circle does not project as the center of the latter.
- 74. Projection of a small circle centered at Y
- 75. Rotation of a pole A1 along an inclined axis B1: B1 B3 B2 B2 B3 B1 A1 A1 A2 A3 A4 A4 A plane not passing through the center of the reference sphere intersects the sphere on a small circle which also projects as a circle .
- 76. Rotation of a pole A1 along an inclined axis B1:
- 77. A 1 rotate about B 1 forming a small circle in the reference sphere, the small circle projects along A 1 , A 4 , D, arc A 1 , A 4 , D centers around C (not B 1 ) in the projection plane
- 78. Rotation of 3 directions along b axis
- 79. Rotation of 3 directions along b axis
- 80. Rotation of 3 directions along b axis
- 81. Standard coordinates for crystal axes
- 82. Standard coordinates for crystal axes
- 83. Standard coordinates for crystal axes
- 84. Standard coordinates for crystal axes
- 85. Projection of a monoclinic crystal +C -b +b -a + a x x 011 0-1-1 01-1 0-11 -110 -1-10 110 1-10
- 86. Projection of a monoclinic crystal
- 87. Projection of a monoclinic crystal
- 88. Projection of a monoclinic crystal
- 89. (a) Zone plane (stippled) (b) zone circle with zone axis ā, note [100] • [0xx]=0
- 90. Location of axes for a triclinic crystal: the circle on net has a radius of along WE axis of the net
- 92. Zone circles corresponding to a, b, c axes of a triclinic crystal
- 93. Standard projections of cubic crystals on (a) (001), (b) (011)
- 94. d/(a/h)=cos , d/(b/k)=cos , d/(c/l)=cos h:k:l=a cos : b cos : c cos measure 3 angles to calculate hkl
- 95. The face poles of six faces related by -3 axis that is (a) perpendicular (b) oblique to the plane of projection